By Melanie Landon-Hays and Tracy L. Smiles
First, a confession. As “literacy people” we are not particularly fond of math. Cultural models are the story lines, or theories that belong to socioculturally defined groups of people (Holland & Quinn, 1987; Strauss & Quinn, 1998; Gee, 1998). We, who identify as “literacy people,” cannot deny the storylines we internalized about math: it is difficult to understand, comes easier for people who are predisposed with mathematical talent, is irrelevant, a set of skills we will rarely use in the real world. Recently, however, through our work with math teachers and our scholarship into inquiry and sign systems, we have been challenged to reconsider our positions, and reexamine the cultural models that have been a part of our literate identities.
| MATH | LITERACY | |
| Involve multiple higher level cognitive skills | Estimation, justification, etc. | Prediction, confirmation, etc. |
| Are linked to identity | I am/am not good at math.” “I do/don’t like math.” | “I am/am not a good reader/writer.” “I do/don’t like to read/write.” |
| Sign systems | Representation through Symbols | Application of Language |
| Ways of making meaning | Reasoning & Proof | Inquiry |
| Connections | Math to Self, Math to Math, Math to World | Text to Self, Text to Text, Text to World |
| Process oriented | Problem Solving | Interpretation and Response |
| Core life skills | Communication | Communication, Expression, |
| Developmental | Over Generalization of processes to concrete understanding of calculations to general understanding of calculations | Recognize and produce sounds (phonemic awareness), recognize difference between grammatical awareness and meaning (over generalize grammatical rules), Grammatical awareness |
| Can be researched through analysis of processes | Error Analysis, Student Error Analysis | Miscue Analysis, Retrospective Miscue Analysis |
| Figure 1.1- Math and Literacy. Harrington, R., Lejeune, M., & Smiles, T. with Clark, P. and Landon-Hays, M. (2009, 2014) | ||
Specifically, our collaborative inquiries have made us realize that literacy and math are more similar than different. The following table reflects an analysis that we, along with colleagues and teachers in Oregon, created as we explored the potentials of math and literacy when integral to each other, and part of a broader system for meaning making. In short, we needed to revise and expand our stories of math.
In fact, we have come to realize mathematics is an important discipline in which to consider global stories. Numbers are often considered international symbols, representing language that is not constrained by the written symbols indicative of what is spoken and read in foreign languages. Numbers represent similar concepts across cultures. They signify amounts and operations that are essential to a unique system of knowing that is often on the opposite pole of the kinds of thinking and learning used in the traditional literacy domain of language arts. In fact, literacy research in content area learning has often misrepresented or neglected the unique nature of mathematical learning. Draper and Siebert (2008) contend:
In particular, literacy messages often do not adequately acknowledge the discipline-specific texts, literacies, and discourses that are an integral part of learning, teaching, and doing mathematics. They also seldom address the “reading” and “writing” of objects that are not traditional print material, or the discipline-specific ways these objects (or even traditional print materials) are used to create, convey, and negotiate mathematical meaning. As a result, it is likely that mathematics educators will find many content-area literacy messages either somewhat irrelevant to their mathematics teaching, or in direct contradiction to the instructional goals and practices that are valued in their discipline. What mathematics educators need are messages that provide them with ways to conceptualize and facilitate the reading and writing of discipline-specific texts, particularly the types of texts that are already present in many mathematics classrooms, in discipline-appropriate ways.
Bearing these fundamental differences in mind, it is important to consider the stories of the discipline of math to not only tell how thinking and learning are facilitated in math, but to use the language and representations of this discipline to promote fluency in these stories. One area which has great potential at providing a critical focus for these stories is in relating the global economic practices, encouraging students to consider the ways that the numbers and symbols they are familiar with translate across cultures to allow average citizens to be economic participants.
Recently, we began collaborating with Phil, a math teacher at an alternative school for teen mothers here in Oregon. While Phil is a content specialist in math, he has chosen to focus on literacy in his graduate studies, demonstrating for us the integral connection between literacy and math. In a course on literacy instruction and assessment, Phil undertook the dilemma he felt as he observed math teachers assessment of student performance as limited to “right” or “wrong.”
He explains, “Educators in the field of mathematics have a special burden that is very different from any other field. Literacy skills apply similarly to language arts, social studies and sciences because these subjects share the same base language. The base language of mathematics is mathematics. Though the base language of mathematics is different than English the concept of literacy still applies; mathematical literacy requires the ability to read and compose calculations. It is undeniable that mathematics has a language it is simply structured differently; instead of sentences and phrases there are equations and expressions; instead of nouns and verbs there are values and operators. Even though mathematics functions as a language it is fundamentally different than other languages in both convention and purpose. Due to these differences the strategies used by reading specialists must be substantially altered if they are to apply to mathematics effectively.”
For example, in order to understand and support his students he created a protocol that, in essence, interpreted stories of math (not to be confused with “story problems”), stories that connected students’ knowledge, experiences, and beliefs to mathematical thinking and performance. Phil’s protocol, similar to miscue analysis, provided an opportunity to systematically analyze students’ processes, and to engage students in analyzing their processes as well. He notes, “One of the most powerful tools in a reading specialist’s inventory is the informal reading inventory (IRI). When conducting an IRI a reading specialist sits with an individual student to observe them read aloud a specially selected reading passage… The value to the educator of hearing a student process text aloud is incalculable in designing individualized instruction to fit the needs of that specific student.”
This protocol, he explains, “attempts to illuminate the process a student goes through as they solve a math problem.” In short, the protocol tells the story of the discipline and the student, as the following example, told in Phil’s words, shows.
“I determined I need further information regarding student C’s lack of comprehension of basic math operations would affect their ability to solve simple pre-algebra problems. I designed an assessment where student C was asked to communicate in writing the mathematical procedures in a problem that had been solve correctly.”
In the third step where student C responded, “She added the 12 and the 4 and got 16,” student C showed a clear lack of understanding of exponent rules. A correct response should include an explanation of how the quantity in the parenthesis was taken to the second power and the result was 16. In student C’s independent calculation a similar error occurs. This shows a basic mathematical misunderstanding, but it also demonstrates a lack of understanding of text structure. If the 12 had been used in step three it would have be eliminated and not present to be used in step five.
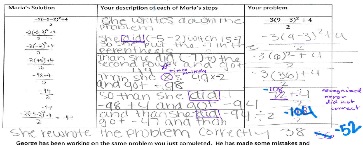
In the second assessment, Student C described step 3 correctly with the correct use of exponents. Student C choose not to use the text marking strategy. When asked, Student C explained that she forgot she could do that. Student C’s original composition contains an error in the fourth step where they multiplied by two instead of three. Student C noticed the error during the assessment, but chose not to correct it. Student C evaluated their performance in light of their goals and stated that they had improved at exponents and organization, but still needed to work on error recognition. I asked student C to add neatness and vocabulary to their goals. Their next attempt is shown below in Figure 5.
Student C has begun to use the marking strategy partially. Additionally, student C has improved neatness of description. The errors in this problem still involve exponents, however the processing has improved significantly. Student C incorrectly multiplied by the coefficient of the second parenthesis before applying the exponent. This is a fairly common mistake when students are learning order of operations. My current plan is to build a word bank for mathematical operations with students to improve students’ use of precise vocabulary.”
Too often math educators dismiss student errors as simply “wrong,” yet through this careful examination, Phil was able to reconstruct his student’s experience through her particular story, and in turn tell a story of math reasoning and development. Much like an analysis of errors in an Informal Reading Inventory reframes them as miscues, this reframing of student reasoning as a process rather than product in Phil’s math classes has empowered Phil to use his assessment data as “instructive evaluation”. This, in contrast to a formative assessment which would benefit the teacher but usually not the student, or as an even less beneficial summative assessment allowing for no revision or reframing for process. Through instructive evaluation Phil is able to collaborate with his students, co-construct their stories in math, and help them to become empowered as authors of their thinking, demonstrating fluency not only of the language of mathematics but the processes as well. He helps them to label their processes in a way that is familiar with stories of other disciplines. Thus, students who are aware of the processes and language of math can transmediate their own learning. They can call on their heightened awareness of a broader conception of both language and process, connecting this learning through a growth in their perspectives contributing to a more ready embrace of inquiry, which is at the heart of global learning.
We feel our exploration of math within the frame of global inquiry through the narratives of math has only just begun, as we are still grappling with these concepts ourselves. However, we are ready to embrace the possibilities of math as an integral part of global inquiry, as we seek to expand our perspectives as learners and as global citizens.
Our last blog will explore the narratives of Social Studies. Meanwhile, we are eager to hear from literacy, content literacy, or math educators on how narrative of math inform your practice and research.
For the full text of the article quoted above, see:
Siebert, Daniel and Jo Draper, Roni (2008) ‘Why Content-Area Literacy Messages Do Not Speak to Mathematics Teachers: A Critical Content Analysis’, Literacy Research and Instruction, 47: 4, 229 — 245.
Journey through Worlds of Words during our open reading hours: Monday-Friday, 9 a.m. to 5 p.m. and Saturday, 9 a.m. to 1 p.m. To view our complete offerings of WOW Currents, please visit archival stream.
- Themes: Melanie Landon-Hays, Tracy Smiles
- Descriptors: Debates & Trends, WOW Currents